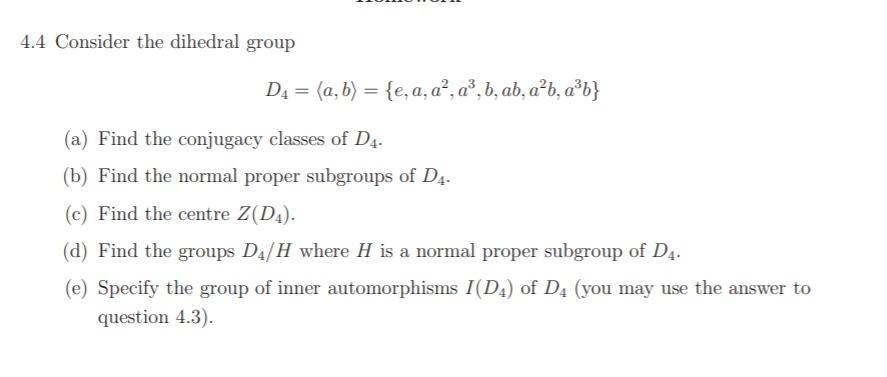
Find the Center of the Dihedral Group D4

Who are the experts. Tˆ3g are all distinct elements of D 4.

Abstract Algebra Drawing Subgroup Diagram Of Dihedral Group D4 Mathematics Stack Exchange

Center of dihedral groups.

. See the answer See the answer See the answer done loading. Abstract Given any abelian group G the generalized dihedral group of G is the semi-direct product of C 2 1 and G denoted DG C 2 n ϕ G. Consider a dihedral group D4 1Find all the conjugacy classes of D4.

Elements in the Center commute with all other. Well look at the general case of D n for n 3. Let D_8 be the dihedral group of order 8.

The groups DG generalize the classical dihedral groups as evidenced by the isomor-. The center of the quaternion group Q8 1 1 i i j j k k is 1 1. In mathematics a dihedral group is the group of symmetries of a regular polygon which includes rotations and reflections.

In the d4-silane matrix the ν4 at 683 cm1 was noticed too. Experts are tested by Chegg as specialists in their subject area. This problem has been solved.

For even n 4 the center consists of the identity element together with the 180 rotation of the polygon. Text Prove that the quaternion group is not isomorphic to the dihedral group D_4. If ρ is such that ρ 4 e look at ρ 2.

Center of Dn contains only 1 if n is odd. We will look at elementary aspects of dihedral groups. Prove that the quaternion group is not isomorphic to the dihedral group D 4.

Contemporary group theorists prefer D 2 n over D n as the notation for the dihedral group of order 2 n. We review their content and use. Dihedral groups are among the simplest examples of finite groups and they play an important role in group theory geometry and chemistry.

All but D3 and D4 which are not sequenceable and perhaps Dbk for k 3 13. Let and let be the dihedral group of order Find the center of. A Write the Cayley table for D 4.

Although this notation is overly explicit it does help to resolve the ambiguity with the Lie type D l which corresponds to the orthogonal group Ω 2 l q. Centralizer Normalizer and Center of the Dihedral Group D_8. For all integers Now since and together.

In this case we are looking for D4 so the number of elements in center will be 2 which is 1 and r2. D 4 D 4 e ˆ ˆ2 ˆ3 t tˆ tˆ2 tˆ3 e e ˆ ˆ 2ˆ3 t tˆ tˆ tˆ3. This can be shown using the formula S n τ n σn.

The center consists of the identity and r5 where r is a frac110 rotation. The homomorphism ϕ maps C 2 to the automorphism group of G providing an action on G by inverting elements. The join of abelian subgroups of maximum order the Thompson subgroup is the whole group dihedral groupD8 so its center is.

Give the detail of your work 2. Show transcribed image text Expert Answer. Find the center of the Dihedral group D4.

For example D4 and D7 have ten subgroups. Our investigations suggest that this radical is formed via radiolysis of silylsilylene H3SiSiHX1A and disilene H2SiSiH2X1Ag. Dihedral group D 4 1.

Using the generators and relations we have. Let S 0 and ˆ R 2ˇn. Where is an element of order 2 is an element of order and are related by the relation It then follows that and in general.

Tˆt ˆ 1 be the dihedral group. Sequencings are related to especially effective shuffles of a deck of cards. Listing its elements relations between rotations and re ections the center and conjugacy classes.

ZD10 e r5 This generalizes to ZDn e rn2 for n is even. You may use the fact that feˆ. We can picture this through a smaller even dihedral group such as D4 shown below.

Let G be a finite group with center Z G if the quotient G Z G is cyclic then G is abelian. D_8langle rs mid r4s21 srr-1srangle aLet A be the subgroup of D_8 generated by r that is A1rr2r3. The elements of D n are 1ˆˆ2ˆn 1 and ˆˆn 1.

We obtain that the only possible cardinalities for Z D 4 are 2 or 1. S11MTH 3175 Group Theory ProfTodorov Quiz 4 Practice Solutions Name. Abelian and any element acting quadratically on it acts linearly on it roughly speaking In a group of nilpotency class two this subgroup coincides with the center.

In geometry Dn or Dihn refers to the. D 4 stands for the group of symmetries of the square. The center of the symmetric group Sn is trivial for n 3.

Discuss all the possible commutators of D4 and. Ections a rotation by a multiple of 2ˇnradians around the center carries the polygon back to itself so D n contains some rotations. Find the center of the Dihedral group D4.

Finding the elements of D n. If n is even it contain 1 and rn2 elements. The center of the dihedral group Dn is trivial for odd n 3.

If or then is abelian and hence Now suppose By definition we have. A Calculate the centre of the dihedral group D 3 the group of sym-metries of an equilateral triangle. Let D 4.

Now you have only to show that theres a non trivial element such that commutes with all the other ones in D 4 but this is very easy Hint. Prove that the quaternion group is not isomorphic to the dihedral group D 4. The number of subgroups of D4 can be represented as S 4 τ 4 σ 4 312410 and S 7 τ 7 σ 7 21710.

B Calculate the centre of the dihedral group D 4 the group of sym-metries of the square. At least three-fourths of the dihedral groups D are sequenceable. Introduction A sequencing of a finite group G is an ordering of its elements xi e x2.

The notation for the dihedral group differs in geometry and abstract algebra. A mass spectrometric study of pyridine N-oxide and pyridine-d5 N-oxide was carried out with a hybrid quadrupoletime-of-flight TOF mass spectrometer coupled with an. The table below demonstrates that there are many dihedral groups that have the same number of subgroups.

Z Dn Centre Of A Dihedral Group D4 Abstract Algebra Group Theory Youtube